
3 X 5 Y 2 2 X Y 4 3y By Substitution Method Brainly In
Algebra Calculator is a calculator that gives stepbystep help on algebra problems See More Examples » x3=5 1/3 1/4 y=x^21 Disclaimer This calculator is not perfect Please use at your own risk, and please alert us if something isn't working Thank youAnswer (1 of 3) 2x3y=6 equation 1 2x3y=15 equation 2 substitute eq1 in eq2 then it comes 6 != 15 ie 6 is not equal to 15 hence x and y has no solution
3-(x-5)=y+2 2x+y)=4-3y by elimination method
3-(x-5)=y+2 2x+y)=4-3y by elimination method-So let's do that We're going to replace y with 5 plus x So this 9x plus 3y equals 15 becomes 9x plus 3 times y The second equation says y is 5 plus x So we're going to put 5 plus x there instead of a y 3 times 5 plus x is equal to 15 And now we can just solve for x We get 9x plus 3 times 5 is 15 plus 3 times x is 3x is equal to 15Then substitute the result for that variable in the other equation 3x2y=12,xy=5 3 x 2 y = 1 2, x y = 5 Choose one of the equations and solve it for x by isolating x on the left hand side of the equal sign Choose one of the equations and solve it for
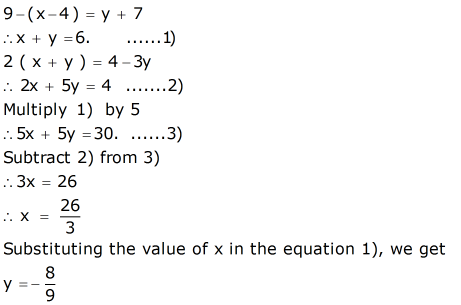
9 X 4 Y 7 2 X Y 4 3y Mathematics Topperlearning Com 5inhk66
Answer (1 of 2) The above ODE shows a linear equation which can be solved using Integration Factor Method xy' 3y =2x^5 Divide through by x (xy')/x (3/x)y =(2x^5)/x y' (3/x)y =2x⁴ IF=e^ln(3/x)=3/x Thus, IF=3/x IFy=∫2x⁴IF 3y/x =∫(2x⁴ 3)/x 3y/x =∫6x³ 3y/x=(6x⁴)/4 c Explanation by appling the laws of exponents ∙ x(am)n = a(m×n) ← (1) ∙ xam ×an = a(mn) (1) is extended to include all factors inside the parenthesis ⇒ (5x2y3)2 = 5(1×2) ×x(2×2) ×y(3×2) × × × × = 52 × x4 × y6 = 25x4y6 ⇒ (2x3y4)3 = 2(1×3) ×x(3×3) ×y(4×3) × × × × = 23 × x9 × y12 = 8x9y12Solve by matrix method 2x 3y 3z = 5 x 2y z = 4 3x y 2z = 3
Simple and best practice solution for 2(x4y)3(2x3y)= equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homeworkExample 6 Rewrite the expression x2y z5 with the last three terms enclosed in parentheses preceded by a minus sign x2yz5=x(2yz5) 23 Multiplication of Polynomials When multiplying monomials in which the variable x appears, we obtain products of the form x^(m)x^(n)The total number of factors of x in this product is m n, so that we have the following law of exponentsX2y=2x5,\xy=3 5x3y=7,\3x5y=23 x^2y=5,\x^2y^2=7 xyx4y=11,\xyx4y=4 3x^2=y,\x1=y xy=10,\2xy=1 systemofequationscalculator y = 3x 5, y = 2x 5 en
3-(x-5)=y+2 2x+y)=4-3y by elimination methodのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 3 X 5 Y 2 2 X Y 4 3y Brainly In | 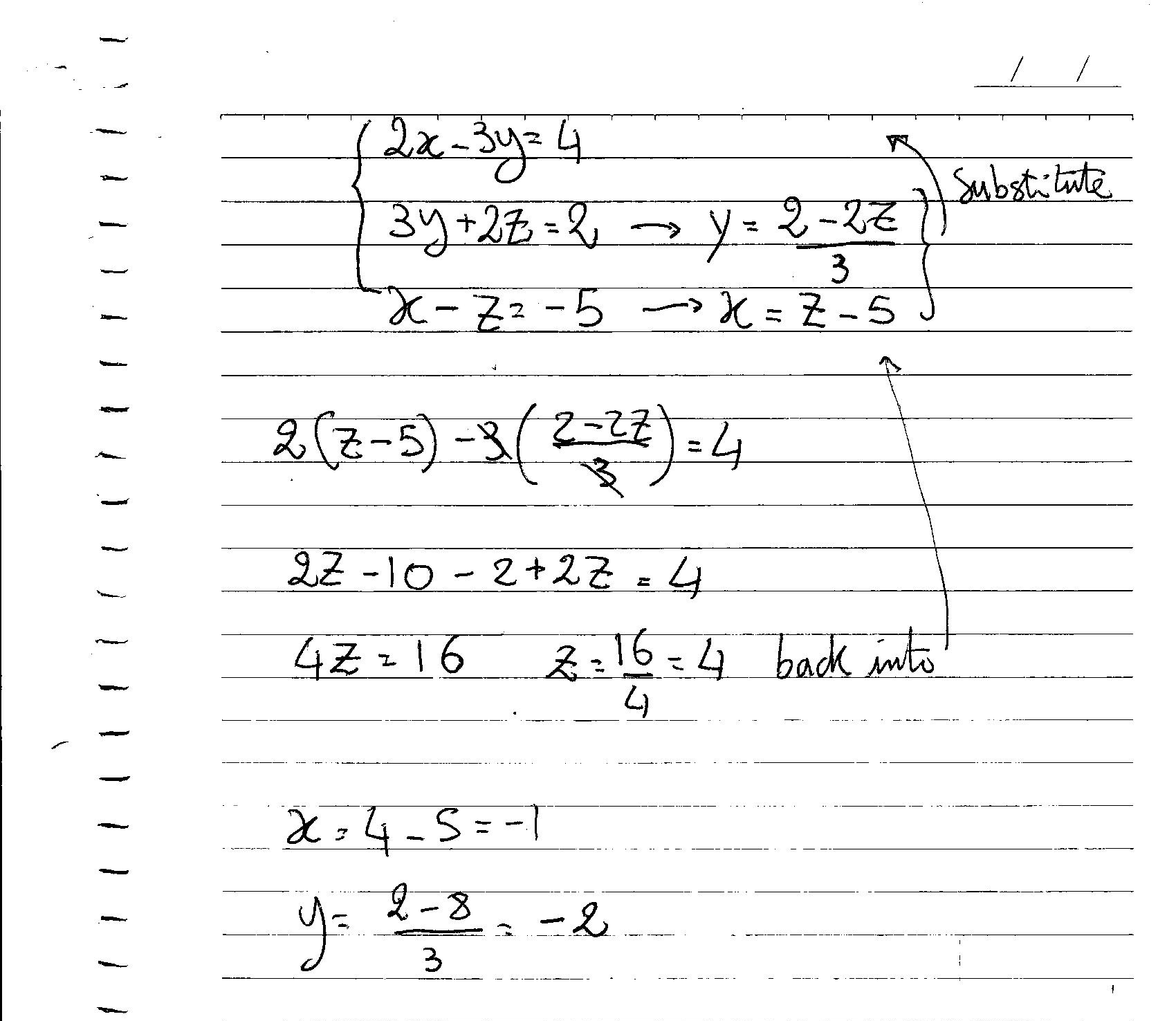 3 X 5 Y 2 2 X Y 4 3y Brainly In | 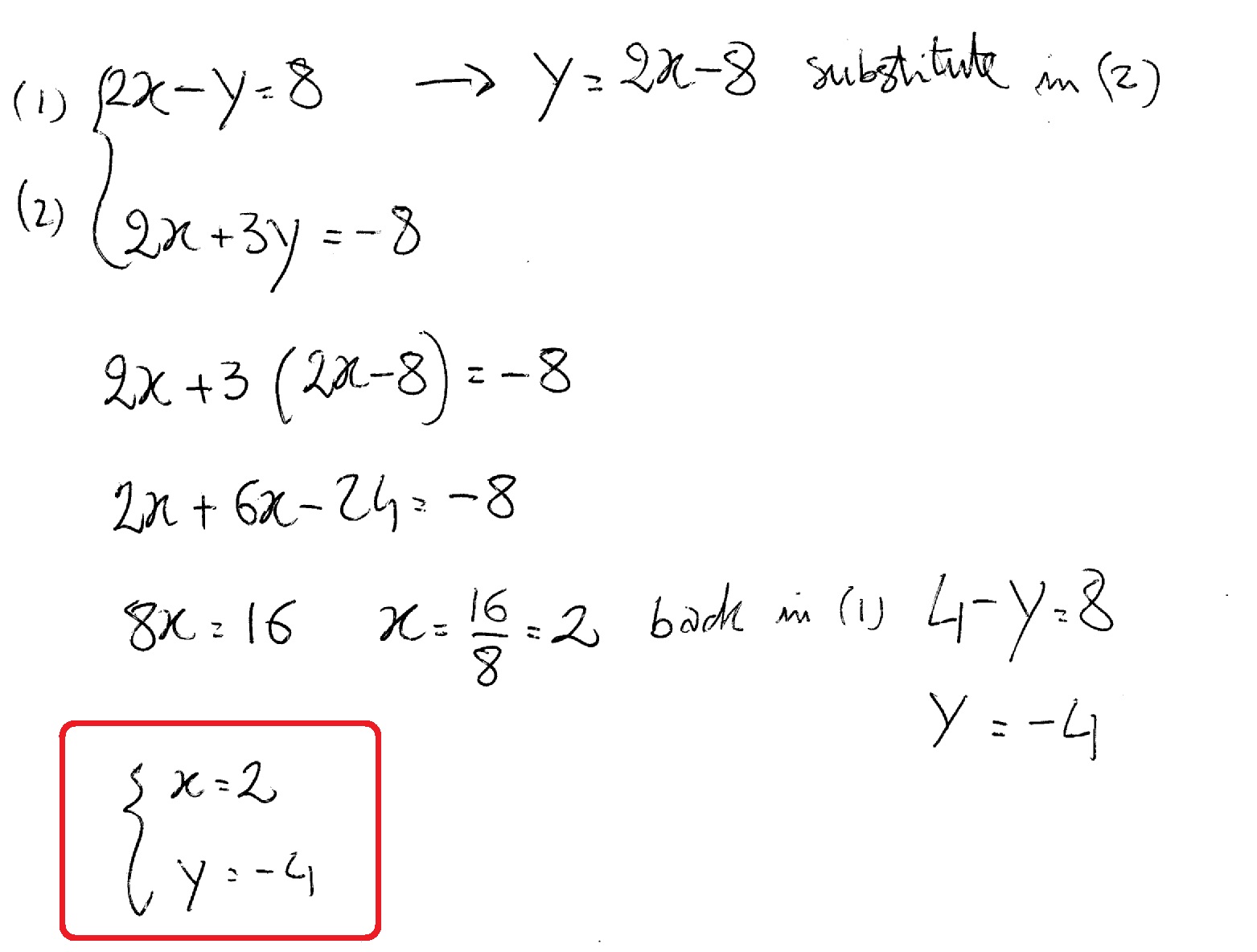 3 X 5 Y 2 2 X Y 4 3y Brainly In |
 3 X 5 Y 2 2 X Y 4 3y Brainly In | 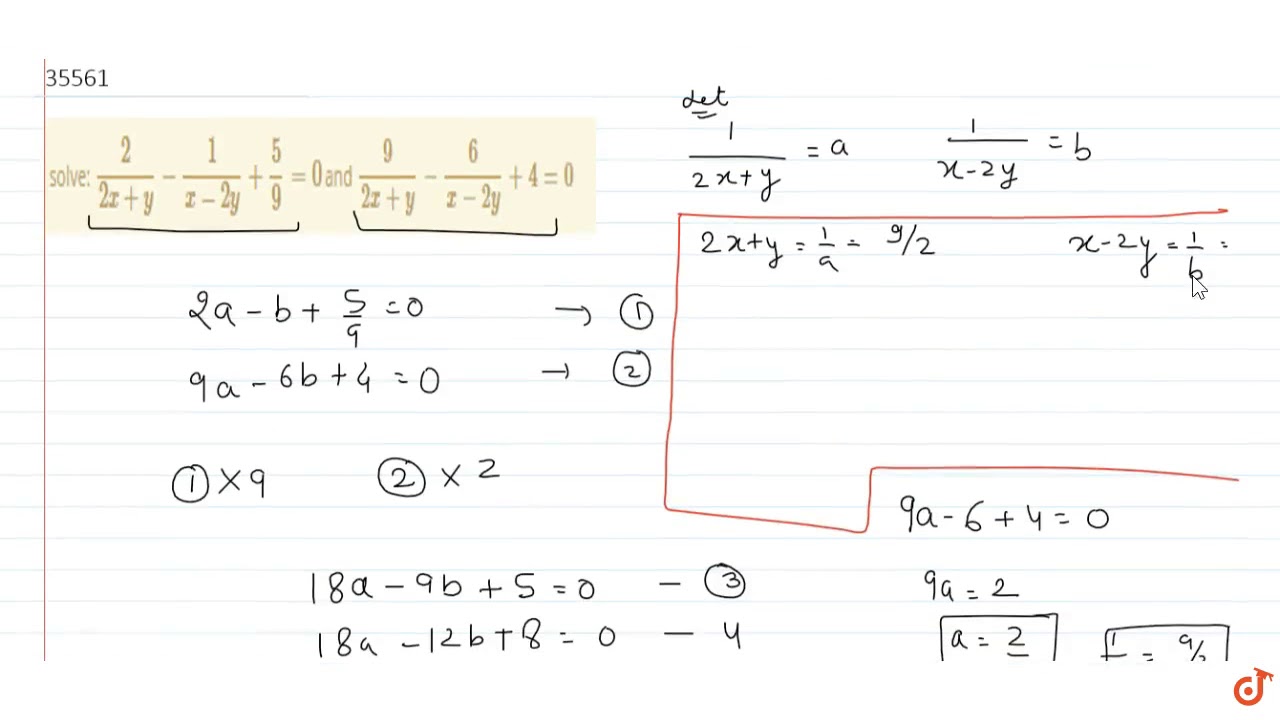 3 X 5 Y 2 2 X Y 4 3y Brainly In | 3 X 5 Y 2 2 X Y 4 3y Brainly In |
 3 X 5 Y 2 2 X Y 4 3y Brainly In | 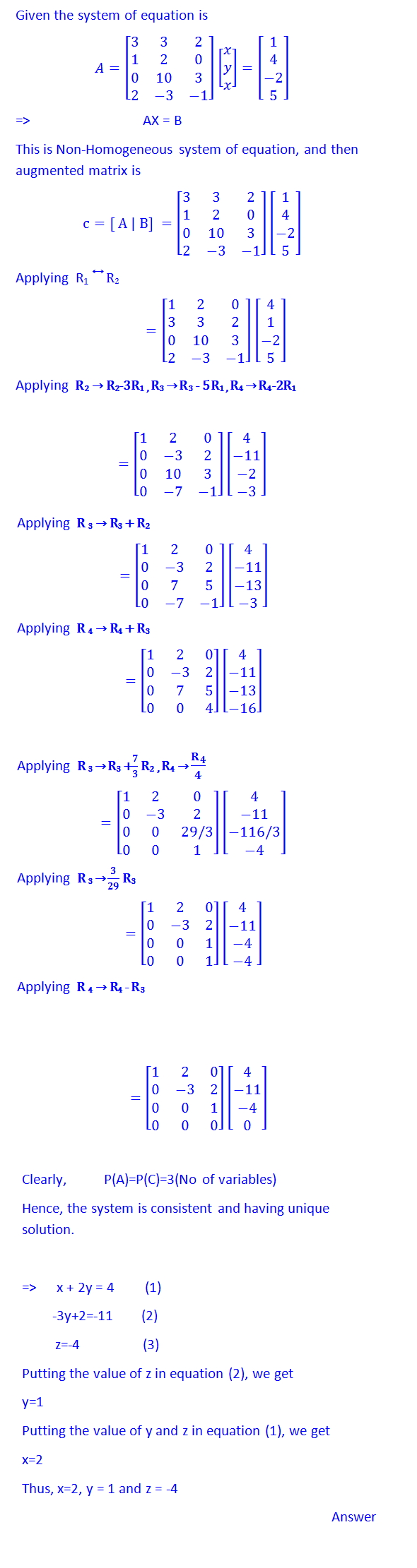 3 X 5 Y 2 2 X Y 4 3y Brainly In |  3 X 5 Y 2 2 X Y 4 3y Brainly In |





0 件のコメント:
コメントを投稿